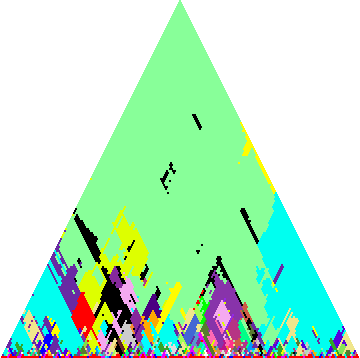
Barbosa 1983
Note: To load data table give above into Excel,
copy and paste the data into a text editor (such as WordPad) first,
then copy the text in the editor and past into Excel. You should
remove the "target" line from the data before pasting into Excel
so that plotting graphs of the data is done properly.
Column descriptions
- Performance:
- 0-Rank/0-Score: 0-Score is equivalent to Pearson correlation
of the entire data sequence between the reference performance and
a test performance. 0-Rank is the sorting order of the 0-scores
(highest score has a rank of 1).
- 1-Rank/1-Score: 1-Score is the area fraction covered by a particular performance in the scape plot (see image above). These values should
not be taken literally, since they are sensitive to the Hatto Effect.
- 2-Rank/2-Score: 2-Score values are equivalent to 1-Score values
with all higher-ranking performances removed before the calculation of
the area of coverage in the scape is calculated. Improvment over the
1-Rank scores, but still somewhat sensitive to the Hatto Effect.
- 3-Rank/3-Score: Similar to 2-Rank calculations. The bottom
1/2 of the 2-rank performances are kept constant as a noise floor for
the similarity measurement. Then one-by-one the top 1/2 of the 2-rank
performances are superimposed with the noise-floor performances, and
a 3-score is measured as the area covered in the scape. This measure
is not sentisive to the Hatto Effect.
- 3R-Rank/3R-Score: Reverse 3-rank/3-scores. 3-rankings and
scores are not symmetric (A->B values are different from B->A values).
So this column represents similarity measures in the opposite direction.
- 4-Rank/4-Score: The geometric mean between 3-scores and
3R-scores. This column gives the best overall similarity ranking
between the various performances (see color codes below).
- NED: Noise Equivalient Distance (not yet implemented)
Color codes for 3-rank listings:
- red = strongly similar performance to target
- orange = moderately similar performance
- yellow = weakly similar performance
- green = marginally similar/dissimilar performance
- white = dissimilar to target
- blue = false positive (has high 3-rank score but low 3R-rank score)
3-rank/scores are not symmetric, so the 3R-rank/score columns
give the 3-rank/scores going in the opposite direction. More matches
in the 3-rank column than in the 3R-rank column indicates an individualistic
performance, while more matches in the 3R-rank column indicates a mainstream
performance.
If a 3-rank and a 3R-rank are both marked as similar to each other,
then there is a possible direct relation between the performances. If one
is similar to the other but not in the reverse direction, then the similarity
is more likely to be by chance (performers randomly chose a similar
interpretation).
|



